


Next: About this document
Up: No Title
Previous: 1.4 lecture note
(PDF ファイル: bib.pdf)
- 1
- G.-Q.Chen.
Convergence of the Lax-Friedrichs scheme for isentropic gas
dynamics (
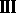 ). Acta Mathematica Scientia 6(1986),
75-120 (Chinese edition: 8(1988), 101-134).
). Acta Mathematica Scientia 6(1986),
75-120 (Chinese edition: 8(1988), 101-134).
- 2
- G.-Q.Chen.
The compensated compactness method and the system of isentropic
gas dynamics. Mathematical Sciences Research Institute,
Berkeley, 1990.
- 3
- G.-Q.Chen.
The method of quasidecoupling for discontinuous solutions to
conservation laws. Arch. Rational Mech. Anal. 121
(1992), 131-185.
- 4
- G.-Q.Chen and J.Glimm.
Global solutions to the compressible Euler equations with
geometrical structure. Comm. Math. Phys.180(1996),
153-193.
- 5
- G.-Q.Chen and P.T.Kan.
Hyperbolic conservation laws with umbilic degeneracy I.
Arch. Rational Mech. Anal. 130(1995), 231-276.
- 6
- G.-Q.Chen, C.D.Levermore and T.P.Liu.
Hyperbolic conservation laws with stiff relaxation terms and
entropy. Comm. Pure Appl. Math.47(1994), 787-830.
- 7
- G.-Q.Chen and T.P.Liu.
Zero relaxation and dissipation limits for hyperbolic conservation
laws. Comm. Pure Appl. Math. 46(1993), 755-781.
- 8
- G.-Q.Chen, D.Wang.
Convergence of shock capturing schemes for the compressible
Euler-Poisson equations. Comm. Math. Phys.179(1996),
333-364.
- 9
- K.N.Chueh, C.C.Conley and J.A.Smoller.
Positively invariant regions for systems of nonlinear diffusion
equations. Indiana Univ. Math. J. 26(1977), 373-392.
- 10
- B.Dacorogna.
Weak continuity and weak lower semicontinuity of Non-linear
functionals Lecture Note in Math.922, Springer, 1982.
- 11
- X.Ding, G.-Q.Chen, and P.Luo.
Convergence of the Lax-Friedrichs scheme for isentropic gas
dynamics (
 )-(
)-(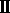 ). Acta Mathematica Scientia 5(1985),
415-432, 433-472 (Chinese edition: 7(1987), 467-481;
8(1988), 61-94).
). Acta Mathematica Scientia 5(1985),
415-432, 433-472 (Chinese edition: 7(1987), 467-481;
8(1988), 61-94).
- 12
- X.Ding, G.-Q.Chen and P.Luo.
A supplement to the papers 'Convergence of the Lax-Friedrichs
scheme for isentropic gas dynamics (
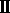 )-(
)-(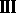 )'. Acta
Mathematica Scientia 9(1989), 43-44.
)'. Acta
Mathematica Scientia 9(1989), 43-44.
- 13
- X.Ding, G.-Q.Chen and P.Luo.
Convergence of the fractional step Lax-Friedrichs scheme and Godunov
scheme for the isentropic system of gas dynamics.
Comm. Math. Phys. 121(1989), 63-84.
- 14
- R.J.DiPerna.
Convergence of approximate solutions to conservation laws.
Arch. Rational Mech. Anal. 82(1983), 27-70.
- 15
- R.J.DiPerna.
Convergence of viscosity method for isentropic gas dynamics.
Comm. Math. Phys. 91(1983), 1-30.
- 16
- L.C.Evans.
Weak convergence methods for nonlinear partial differential
equations CBMS regional conference ser. in Math. 74, AMS, 1990.
- 17
- E.Feireisl.
Time-periodic solutions to quasilinear telegraph equations with
large data. Arch. Rational Mech. Anal. 112(1990),
45-62.
- 18
- M.Kru
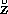
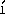 k.
Explicit characterization of
k.
Explicit characterization of 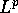 -Young measures.
J. Math. Anal. Appl. 198(1996), 830-843.
-Young measures.
J. Math. Anal. Appl. 198(1996), 830-843.
- 19
- P.Lin.
Young measures and an application of compensated compactness
to one-dimensional nonlinear elastodynamics.
Trans. Amer. Math. Soc. 329(1992), 377-413.
- 20
- P.L.Lions, B.Perthame and E.Tadmor.
Kinetic formulation of the isentropic gas dynamics and p-systems.
Comm. Math. Phys. 163(1994), 415-431.
- 21
- T.Makino and S.Takeno.
Initial boundary value problem for the spherically symmetric motion
of isentropic gas. Japan J. Indust. Appl. Math. 11(1994),
171-183.
- 22
- P.Marcati and R.Natalini.
Weak solutions to a hydrodynamic model for semiconductors and
relaxation to the drift-diffusion equation.
Arch. Rational Mech. Anal. 129(1995), 129-145.
- 23
- P.Marcati and R.Natalini.
Global weak entropy solutions to quasilinear wave equations
of Klein-Gordon and Sine-Gordon type. preprint (1996), 1-23.
- 24
- J.M
 lek, J.Ne
lek, J.Ne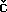 as, M.Rokyta and M.R
as, M.Rokyta and M.R
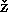 i
i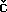 ka.
Weak and measure-valued solutions to evolutionary PDEs. Chapman &
Hall, London, 1996.
ka.
Weak and measure-valued solutions to evolutionary PDEs. Chapman &
Hall, London, 1996.
- 25
- J.A.Nohel, R.C.Rogers and A.E.Tzavaras.
Weak solutions for a nonlinear system in viscoelasticity.
Comm. Partial Differential Equations 13(1988), 97-127.
- 26
- B.Rubino.
Compactness framework and convergence of Lax-Friedrichs and
Godunov schemes for a
 nonstrictly hyperbolic system
of conservation laws. Quart. Appl. Math. 53(1995),
401-421.
nonstrictly hyperbolic system
of conservation laws. Quart. Appl. Math. 53(1995),
401-421.
- 27
- W.Rudin.
Real and complex analysis. McGraw-Hill, New York 1996.
- 28
- M.E.Schonbek.
Convergence of solutions to nonlinear dispersive equations.
Comm. Partial Differential Equations 7(1982), 959-1000.
- 29
- D.Serre.
La compacit
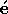 par compensation pour les
syst
par compensation pour les
syst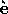 mes hyperboliques non
lin
mes hyperboliques non
lin aires de deux
aires de deux  quations a une
dimension d'espace. J. Math. Pures Appl. 65(1986),
423-468.
quations a une
dimension d'espace. J. Math. Pures Appl. 65(1986),
423-468.
- 30
- D.Serre.
Syst
 mes de lois de conservation
mes de lois de conservation  ,
,  Diderot,
Paris 1996.
Diderot,
Paris 1996.
- 31
- J.Shearer.
Global existence and compactness in
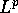 for the quasi-linear
wave equation. Comm. Partial Differential Equations
19(1994), 1829-1877.
for the quasi-linear
wave equation. Comm. Partial Differential Equations
19(1994), 1829-1877.
- 32
- J.Smoller.
Shock waves and reaction-diffusion equations. (2nd edition),
Springer, 1991.
- 33
- S.Takeno.
Initial boundary value problem for isentropic gas dynamics.
Proc. Royal Soc. Edinburgh 120A(1992), 1-23.
- 34
- S.Takeno.
Free piston problem for isentropic gas dynamics.
Japan J. Indust. Appl. Math. 12(1995), 163-194.
- 35
- S.Takeno.
A time-periodic solution for a scalar conservation law.
preprint (1996), 1-26.
- 36
- L.Tartar.
Compensated compactness and applications to partial differential
equations. 136-211, Nonlinear analysis and mechanics:
Heriot-Watt Symposium, Vol.4, ed. R.J.Knops, Research Notes in
Mathematics 39, Pitman, London 1979.
- 37
- L.Tartar.
The compensated compactness method applied to systems of
conservation laws. 263-285, Systems of nonlinear partial
differential Equations, ed. J.M.Ball, NATO ASI Series, 1983.
- 38
- B.Zhang.
Convergence of the Godunov scheme for a simplified one-dimensional
hydrodynamic model for semiconductor devices.
Comm. Math. Phys. 157(1993), 1-22.
- 39
- Y.Zhou.
An
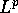 theorem for compensated compactness.
Proc. Royal Soc. Edinburgh 122A(1992), 177-189.
theorem for compensated compactness.
Proc. Royal Soc. Edinburgh 122A(1992), 177-189.



Next: About this document
Up: No Title
Previous: 1.4 lecture note
Shigeharu Takeno
�$BJ?@.�(B9�$BG/�(B5�$B7n�(B1�$BF|�(B (�$BLZ�(B), �$B8a8e�(B 4�$B;~�(B1�$BJ,�(B0�$BIC�(B